Pages
31 December 2008
Blog thoughts
My Candyland post got a lot of attention, and I'm working on a post about Monopoly. Guess what? It's a Markov-chain too (nearly). There is a lot of material available on this (just Google Monopoly game math), but I think I can add something a little different.
Are there games people want to see analyzed? I'm not sure I can take requests like that, but the question itself might be interesting.
Someone asked what other games I've analyzed (maybe over-analyzed), and I'll have to think about that. Probably not all that many in any depth, but I've never made a list (maybe I should).
I've got a series of posts that require a fair bit of spreadsheet work to set up, and a laborious effort to describe so that someone else might be able to figure out what I've done. This is painful to write, and probably more painful to read. This is also my best way to "share the math" in a way which is accessible to others, and really essential to what I am trying to do. I need to find a way to make this type of post work.
The downside of writing a lot is I'm not doing much painting (or much laundry). I need to balance things a bit better, or become a faster/better writer. Of course, writing a lot is the key to becoming a better/faster writer.
There is a big topic I've been trying to work up to writing about: Game Theory. This is another one of those hard to write but important to do things I need to figure out how to do.
What else is in the works?
More miniatures pictures, an interview, a browser game with mecha in it, that long-promised Facebook Games followup, Elo scoring, some old-but-great games in my closet, some new games, the game I'm trying to write, some simple "demonstrate the math" games, not to mention following up on my blogroll and some of you nice people who are following this blog.
So that's a bunch of ideas even at one post each, and some of those need multiple posts. If I could hit on all those topics and do a decent job on them, that should easily keep me busy for a good part of 2009, and that's not counting the other games I find along the way.
So that's a quick rundown of some of the topics coming on GBR, at least the ones running through my head at the moment. Happy 2009, and happy gaming!
PS: 3-4 more ideas since I "finished", but this is enough
30 December 2008
Battle of the Sexes
 Ed Yong has a great post over at Not Exactly Rocket Science. (Seriously, I might have to put it on both my blogs.) The question here is "Are men better than women at Chess?", and by association "Are men smarter than women?". [Image from Corbis]
Ed Yong has a great post over at Not Exactly Rocket Science. (Seriously, I might have to put it on both my blogs.) The question here is "Are men better than women at Chess?", and by association "Are men smarter than women?". [Image from Corbis]The short answer is ...
... no. Don't let that stop you from reading the full post though.
Far more men play chess than women and based on that simple fact, you could actually predict the differences we see in chess ability at the highest level. It's a simple statistical fact that the best performers from a large group are probably going to be better than the best performers from a small one. Even if two groups have the same average skill and, importantly, the same range in skill, the most capable individuals will probably come from the larger group.
The article goes on to discuss Elo scoring as well, something I've mentioned before and will come back to again.
28 December 2008
Why I Hate Computer Games
More than that, I tend to obsess over them. I have had to set some games aside, never playing them again or refusing to buy them in the first place, otherwise I would spend all my time playing or thinking about them. Games in the Civilization, Warcraft/Starcraft series are maybe the most recent examples of this, but there are plenty of others (Doom!, Quake, etc), and the next one is calling to me from underneath the Christmas tree where I stashed it along with other gifts.
I wrote about Gran Turismo 2 a while back, and how I set up spreadsheets to calculate optimal gearing ratios for my cars in the game. This is something a serious gearhead or racer might do, but for a game? Objectively I'd have to say this is just way too much effort spent on a game. On the other hand, I think this is part of how I like to play a game - I really want to understand at a very basic level. In this case I actually learned a lot about the application of physics that I had never really thought about before. I had a lot of fun creating those spreadsheets tinkering with the real world physics as it applies to automobiles, both real and virtual. (This is also a real testament to the physics engine driving games such as Gran Turismo, it's so good you can study the real world by studying the game!)
I also spent a lot of time alone playing and working on Gran Turismo 2 (or Civilization, etc.), and contributing little to my household, my family, and my life in general. Those are some great games, enormous fun and I can play them for hours or days, but they tend to leave me feeling like I've wasted my time when I am done playing. I hate that feeling. Therefore I now budget my time to those games that give me back the most socially, creatively, and intellectually. For me, the games that give back the most are the tabletop games, and that is why I tend to focus on them in this blog.
So not to dis computer games, it's just that I love them too much.
26 December 2008
Guitar Hero Q&A with Alex Rigopulos
Guitar Hero fans (not me I'm afraid but my nieces like it), there is Q&A with Alex Rigopulos at Freakonomics. Video games on a Big Time blog!
Time to Play
Gaming means a lot to me. It's a a social activity, an outlet for creativity, and a subject of study.
I meet regularly with friends for a weekly session of Battletech. It's a mixed groups of working professionals, craftsmen, and students. We are not there for drinkin' an smokin' (maybe a little cussin' tho); we are there to play, and to borrow a phrase; a bad evening gaming is better than a good day working. I am a bit unusual in the group because I don't play a lot of games other than Battletech (I do have limited time for gaming), and most of the guys also play other games on a regular basis. (Note aside: I also like computer games, but I find these do not have the same aspect of social interaction, so I don't play them nearly so much. Thoughts?)
A fair bit of my time (likewise many of the guys) goes into game related activity such as painting and assembling miniatures. Working with miniatures is a hobby within a hobby, and a topic for another day, but I love working with minis as much as I like to play. Maybe more. Check some great galleries of Battletech minis at LOTB, and consider the craftsmanship that goes into some of these.
The other creative aspect of the hobby (for me) is writing, and I don't just mean this blog. I've been writing related to gaming on and off for years. This might be writing up a scenario or adventure, describing something I'd like to represent in a game, writing my own game, or working out strategies. Most if this writing isn't interesting to anyone but myself, but I enjoyed writing it all the same. More recently I actually became somewhat organized about recording my game related ideas, and in the process I realized I had something I wanted to say to others (and so here we are).
Finally, I like to study games. I wrote my first computer simulation program when I was 13 or 14 on my Apple II+. Why? Because I wanted to settle an argument about whether it is better in the game Risk for the defender to roll one die or two (short answer: two whenever possible). Little did I know I was actually taking my first steps towards being a statistician, I just wanted to know the best way to play. More recently I decided to really look hard into the mathematics of the game Battletech. Rather than write a program I decided to try to get into the theory behind it. Part of me wonders why I never really tried to apply my skills this way before, but maybe I wasn't ready? What I quickly learned is that I wasn't just studying Battletech, but the math that applies to a whole class of games, sports, and even military studies. I've always loved games, but until recently I never knew they could be so deep.
So now I've rambled on too long and need to get to work. I invite you to post comments on why you like games, why you play them, and what intrigues you about them. How do you spend your play time?
25 December 2008
Merry Christmas from GBR
 Dashing through the snow,
Dashing through the snow,in a Giant Battle Mecha ...
O'er the tanks we go,
Smashing all the way!
Ok, so maybe I won't give up the day job to become a lyricist, and those missiles are delivering presents, honest!
There have been over 4000 new visitors to this blog since it was posted on Blogs of Note Tuesday, and I am gratified and overwhelmed by the attention. Thanks to everyone who stops by, and thanks to the folks at Blogspot (Santa Blog?, Saint Blogolas? Father Blogmas?) for noticing me.
Merry Christmas everyone, and I hope that one or two of those nicely wrapped boxes under your tree might have a games in them.
23 December 2008
The Mathematics of ... Candyland???
 I mentioned Markov-chains a while back (not to be confused with Markov Chaney), and I though that might make an interesting topic to relate to games. It seems though that
I mentioned Markov-chains a while back (not to be confused with Markov Chaney), and I though that might make an interesting topic to relate to games. It seems though that [Image taken from lscheffer.com, who also has a nice analysis.]
In my own words, a Markov-chain is a series of random states. A trivial example might be flipping a coin; the coin is either in a state of "heads" or "tails" and has a 50% chance of changing state with each flip. It can get more complicated, with the probabilities of changing to a different state dependent on the current or even (correct me if I am wrong) previous states. A state which ends the series (if any) is called an absorbing state. In my recent Netrek post the number of planets a team controls would be the state, and the absorbing state would be one team conquering all the planets, thus ending the series and the game (oversimplified, but true).
Candyland is a Markov-chain if you reshuffle the deck of cards after every draw. Each square (rhomboid?) on the board represents a state, and players advance randomly towards the end of the game. Reshuffling is required so that the probability of changing states remains independent of previous card-draws (if you were wondering). There is a nice summary and history of the game itself here.
Speaking of fine work, Greg Costikyan wrote about this a few weeks back, and it has traveled far in the blogosphere. The really interesting part here is (for me) the discussion of whether or not it really is a game; all moves are random, so if the player doesn't actually do anything is it really a game? My answer: Yes. Not the most interesting game we may ever play by any means, but most definitely a game. Games where players make decisions and enact strategies are more difficult, and for most, more interesting.
 I should not end without mentioning an older game that is a Markov-chain to start with - no reshuffling required - known to most as Snakes and Ladders.
I should not end without mentioning an older game that is a Markov-chain to start with - no reshuffling required - known to most as Snakes and Ladders.[Image from DKimages]
21 December 2008
Convergence of Spheres, Science and Gaming
 If you can picture the number in each hex as a height, making the tallest hex on the map the best move, then we have a representation of the game (the current move) as a sort of fitness landscape. This "landscape" probably looks very different from the Battletech game map, and I think of it as an abstract representation (possibly in many dimensions) of the choices to be made in the game. Basically your best move is always to move towards the highest point in this landscape.
If you can picture the number in each hex as a height, making the tallest hex on the map the best move, then we have a representation of the game (the current move) as a sort of fitness landscape. This "landscape" probably looks very different from the Battletech game map, and I think of it as an abstract representation (possibly in many dimensions) of the choices to be made in the game. Basically your best move is always to move towards the highest point in this landscape.In a simple scenario where each player has one Battlemech it is actually not too hard to picture how such a landscape might look. However, when the scenario is more complex and each side has multiple 'Mechs to move this landscape can get very complicated indeed, and the choice of movement for one unit affects the landscape for all the others. If we want to get really deep, we might think about the entire game as a sort of landscape instead of just a single move.
This representation of a game as a landscape is the "converence" I was getting at in the post title, where a method (or at least the representation) from science has meaning for a game. I've never worked with genetic algorithms myself, but it seems like they might be very well suited to solving "best move" problems in games too. More importantly though, this gave me an opportunity to introduce the concept of a game landscape. Thinking about games in this way is probably an unusual concept for most gamers, but it is incredibly useful to be able to think about a game with the sort of mathematical representation that this allows.
20 December 2008
Fear of Failing

Jesper Juul has a book out, or rather a chapter he wrote which is collected in this book which is coming out soon.
Juul writes:
Winning isn’t everything
It is quite simple: When you play a game, you want to win. Winning makes you happy, losing makes you unhappy. If this seems self-evident, there is nonetheless a contradictory viewpoint, according to which games should be “neither too easy nor too hard”, implying that players also want not to win, at least part of the time. This is a contradiction I will try resolve in what follows.
I read the Fear of Failing article, and recognized his "Snake" game and the accompanying survey. I don't know if was one of his study participants or if I encountered it at some later time, but I recall play this game and completing the survey (some years ago). Now that I'm trying to write about games on my own, it is particularly interesting to have this particular research come back to me in this way.
-----
 But back to the article: Mr. Juul gives an excellent account of the balance between game difficulty and player skill/ability, and that the proper balance is what make a good game. He is writing about video games in particular, but this easily applies to the sort of tabletop games as well.
But back to the article: Mr. Juul gives an excellent account of the balance between game difficulty and player skill/ability, and that the proper balance is what make a good game. He is writing about video games in particular, but this easily applies to the sort of tabletop games as well.Before I forget, the image to the right (© 2004 Noah Falstein, reference: Falstein, Noah. 2005. "Understanding Fun—The Theory of Natural Funativity". In Introduction to Game Development, ed. Steve Rabin, 71-98. Boston:Charles River Media.),
Then I got to thinking, and although I still think this applies to tabletop games, is not so simple to describe what is going on you add in the dynamics of human players, either in tabletop games or multiplayer video/computer games. I touched on this briefly when I wrote about The Mathematics of Netrek, and I intend to spend some more time on this in future posts.
The Science of Dice, with Colonel Louis Zocchi
Part 1:
and Part 2:
Louis Zocchi is a great one ... but now I may have to replace all my dice.
[Update] Critical Gamers (whom I just discovered) have a post on this topic too, and a favorable review of Game Science dice in use.
19 December 2008
Grow Old, Stay Young, Play Games.
Playing Board Games Helps Prevent Degenerative Diseases
Many individual do not realize the benefits of playing board games especially in relation to health. There are certain ways in which board games can be implemented for the prevention of certain degenerative diseases like Alzheimer’s disease and Dementia. Alzheimer’s disease or AD is a fatal and progressive disease. This disease destroys brain cells and leads to memory loss, behavior and thinking, which can affect social work, hobbies and also work. Research was conducted that showed that when the brain gets challenged through leisure activity stimulation, it greatly reduces the chances of contracting this disease.
Click through for the full article
18 December 2008
Conditional probability (sort of)
17 December 2008
Scholeologist Defined
My favorite bit:
When I was young and naive, I came across a brilliantly excitingly named branch of mathematics called "Game Theory". Naturally I said to myself, "holy crap! Pretty darn smart of me to become a mathematician -- now I'll get to play games for a living!Now go read the post at Curmudgeon Gamer!
In case you aren't aware, "Game Theory" is a bait-and-switch ruse right up there with "Greenland".
I confess I had a similar first contact with Game Theory, and even would have signed up for a class has it been offered. Now I've gotten back to the point where it might be interesting again, but that is a tale for another day.
14 December 2008
Chart of Dungeons & Dragons History
 [Click Through to Adventures in Gaming]
[Click Through to Adventures in Gaming]There is a lot of other good material on this blog as well, and I plan to spend some time exploring it.
10 December 2008
Mathematics of Netrek
Player versus Player: One-on-one, ship-to-ship combat (dogfighting) is perhaps the most basic component of Netrek. Players combat each other with phasers and torpedoes, trying to inflict enough damage to destroy the other ship first. There is an aspect of Point Objective Games here, with the added complexity that shields regenerate and ships repair. This complexity aside, it comes down to a contest of skill between players, rather like a Chess match. Chess has a ratings system, Elo Scoring, which is used to describe the past performance of a player, and (ideally) has some usefulness in predicting the winner of a match between two players who have never met before. Elo scoring uses a scale where a 200 point difference between player ratings is interpreted as a 75% chance that the player with the higher rating will win (assuming the ratings accurately represent player skill). Chess is considered to be a very “deep” game, with a very great range or many levels of skill between the worst and best players, and this deepness can be measured (in part) by the range in scores. Beginning Chess players might have a rating of 800-1000 points, while the World Championship Chess players have ratings of 2500 or more (Go has even greater depth by this measure). Netrek also has considerable depth on player skill, and it would be interesting if this sort of ratings system could be implemented to measure it. Mathematics based measurements of player skill in games is one of those topics I intend to discuss at greater length in future posts.
Team versus Team: This is where the game of Netrek really shines. And if we could measure the skill of a “team” with a sort of Elo score in the same way we might measure individual players’ skill, I’m pretty sure the depth of Netrek would approach that of Chess. Tournament mode (T-mode) play in Netrek has an underlying mathematical structure that is actually fairly simple. I want to deconstruct the game and point out where the basic mathematics applies to the team games.
Suppose we have the following simple game: 20 Coins are laid out on a table with 10 heads and 10 tails. [edit: here the coins respresent planets, and each side wants to capture all the planets.] Two players, one represented by heads and the other by tails, each turn both players choose one coin and flip it, with the object of making all the coins either heads or tails (with 50/50 chance). Obviously the heads-player will select any coin showing tails and flip it, trying to get it to come up heads, and the tails player will do the opposite. This game is essential a discrete random walk, and with every turn (one flip for each player) there is a 25% chance a player will gain a coin, 25% chance they will lose a coin, and a 50% chance that things stay the same. This is in concept very close to a completely random T-mode Netrek game. (This might also be stated as a Markov chain.).
A purely random game like this could go on for quite some time (it might even be infinite), but suppose there were some element of skill involved so that one player (lets say heads) is a little bit more likely to get the desired result than the other. So now maybe each turn there is a 35% chance the heads-player will gain one coin, a 20% chance the tails-player will lose one coin, and a 45% chance that things stay the same [edit: both win, or both lose.] Out of every 20 turns we would expect that heads would gain 7 coins (35% of 20) while losing 4 (20% of 20) for a net gain of 3 coins. We should expect the heads player will win in 67 turns, on average.
Netrek takes this same mathematical structure and adds a lot to it (players of varying skill, a variety of ships and planets, etc.). I wonder if it is possible to measure the “team playing” skill of players in the same way as Elo scoring might be used to measure the individual dogfighting skills of players? This would be rather complicated, and likely impractical, but it is interesting to think about.
Resource Allocation: This is the final aspect I want to write about today, and the part that leads to the wonderful complexity of team play in Netrek (It will also be the least mathematical discussion). Resources in this game are not equal; The team with more planets has greater potential to gather armies and try to take planets. More planets than players mean that is it not possible to defend every planet equally. Players are not equally skilled, but have varying levels of ability at individual and team play. Finally, controlling a greater number of planets increases the distance the leading team must travel to front-line-combat, and players are out of position for a longer period of time (though Starbases help).
This last part has an effect not seen in most games; the closer one team is to winning (in an otherwise balanced game) the harder it is to successfully take more planets, because the losing side can respond to defend remaining planets much more quickly – and it tends to make the game self-balancing. I am not a very good Netrek player (I confess to being a twink), and I have never participated in a real “clue” game involving 16 really good players, but my understanding is that in these games an outright victory is rare; most games are given a set time limit where the team in control of the most planets at the end of time is declared the winner. This is entirely consistent with Netrek being a self-balancing game.
One common complaint I recall players making is about how hard it is to actually win a T-mode game. There are many reasons for this, certainly more than I have discussed, but I would propose the following: In a really balanced game with equally skilled players on each side, it may be nearly impossible to actually win, either by Genocide (all planets) or Timer (all but three planets for ~20 minutes). Therefore, most wins probably occur in unbalanced games.
[There is an alternate version of this post, co-authored by Zach Uram, Netrek player extraordinaire, that goes deeper in the game history and mechanics. Give that a read too, and check out Zach's blog at http://www.fidei.org/2011/01/mathematics-of-netrek.html.
09 December 2008
08 December 2008
Ultrabots
Ultrabots: Fairly simple to learn with wireframe graphics (state of the art) and very few glitches. I picked up my copy used in '95-95, played it through and beat it on normal difficulty the first try, then again on hard that same afternoon, at which taught me something about replay value. It was a good game though, if only for a single scenario where I pulled a glorious victory out of an "all is lost" situation. Turns out the AI was entirely too single-minded about its objective, and let my lone operating humanoid bot take out the attacking force in Sergeant York fashion.
06 December 2008
Metal Fatigue
Try this simple experiment: First, take a small piece of metal and try to break it. A straightened paperclip will do nicely - literally try to pull it apart, use pliers to get a good grip if needed, and pull without bending or twisting. This ought to be difficult, and odds are you probably can't break the metal this way barehanded. For the second part of this experiment, bend the metal; work it back and forth several times bending and re-straightening it in the same place. Now pull again, and the metal should part fairly easily. If not, bend it some more and try again, OR just keep bending it until it breaks. Why does the metal, originally quite strong, now break easily?
The answer is metal fatigue (which I previously hinted at here). Metal is malleable and easily bent, and when subjected to stress it develops tiny cracks, The more times the metal is stressed, the longer these cracks become (growing in an essentially random manner). Eventually these cracks reach some critical length and the metal breaks.
Exactly how long a piece of metal can be stressed before it breaks is difficult to predict, but there is a nice mathematical results (Birnbaum and Saunders, 1969) that derives the theoretical probability distribution that describes how long a metal part under repeated stress will last. Furthermore, this is an application of the Central Limit Theorem, and will give a useful approximation to the large sample behavior of survival time in many similar circumstances, and not just for metal parts (exceptions are a topic for another day). So here is the formula that describes the Cumulative Distribution Function (CDF) of the Birnbaum-Saunders distribution for fatigue life:
(It is at this point I realize there may be some difficulty in expressing a formula within the format of a blog without some special software tools. Please forgive the crude scribbles done in Paint.)
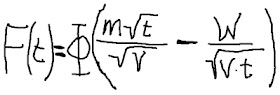 where Xi is the magnitude of the stresses or shocks, Xi > 0, which have mean m and variance V, t (where t>0) is the number of shocks, W is the critical length of a crack that will break the part when the sum of the shocks Xi is greater than W, and the Greek-looking-thingee is the Normal CDF function. I might do as well to present this is Excel-formula-like notation:
where Xi is the magnitude of the stresses or shocks, Xi > 0, which have mean m and variance V, t (where t>0) is the number of shocks, W is the critical length of a crack that will break the part when the sum of the shocks Xi is greater than W, and the Greek-looking-thingee is the Normal CDF function. I might do as well to present this is Excel-formula-like notation:and
F(t) = NORMDIST(Z,0,1,1)
which is very close to something you can plug into an Excel spreadsheet and use**. Lets try this one more time in English: The formula F(t) gives the probability that the part will break on or before some number of shocks (t), or that the sum of t shocks (the Xi's, defined by m and V) will be greater than W. For a less scatterbrained definition of the Birnbaum-Saunders distribution, see this excellent source.
**I will provide a spreadsheet to demonstrate this, but that will get put off to another post so I can get to the point (yes, there is a point to this).
The mathematical theory doesn't care anything about metal, it is describing the probability that the sum of a series of random numbers X will be greater than some number W. This is a Central Limit Theorem result, which means that we can use almost any sort of random numbers, like those generated using dice or cards, as long as we know the mean and variance. Further, if we a playing a point scoring game with some fixed number of points that are needed to win, then we know W. Therefore, we have a mathematical tool that can describe the probability distribution of a Point Objective Game.
Dramatic pause and a deep breath. I have done a fair amount of work to get to this point, and it is going to pay off, because I now have a tool to help analyze a whole class of games. One of these games is Classic Battletech - which I do not deny is a very complex sort of Point Objective Game - but it can be done. I have a number of other topics to cover along the way too, and this is just the beginning.
[Part Two]
05 December 2008
04 December 2008
Why techies love games
By Tom Kaneshige
Everyone knows that techies love "Dungeons & Dragons," where they can prowl the bowels of a castle and cast spells on clueless managers, er, mages. After all, it's just a game.Click through to InfoWorld for an interesting read on the connection between Tech skills and gaming. This article considers computer gaming only, but I think it certainly extends to tabletop games as well.
Or is it?
02 December 2008
Revisit Vintage Games
Les Chappell writes at The Escapist
I've always been behind the curve when it comes to videogames, going back to childhood when my parents refused to buy a Nintendo and my brother and I had to rely on older PC games for entertainment. While I never developed the manual dexterity necessary to win an Xbox deathmatch (my performance in Halo is a joke), I do have an interesting series of childhood recollections: top-down terrorist control in Syndicate, the quirky adventure game-style of Quest for Glory, spinning to avoid giant feline pilots in Wing Commander II.
With the old disks lost or rendered obsolete, I thought these games existed only in memory. That is, until a few months ago when my friend Pat mentioned that he'd been playing through System Shock 2, a game I'd heard many great things about but missed when it came out in 1999. Where'd he find it, I asked - garage sale? eBay?
Click through for the full article, and maybe revisit some old favorites? There is also a lively discussion of abandonwear in the comments.
30 November 2008
Survival Distributions
It is not my intent to turn this blog into a statistics textbook, but there are some topics I feel like I ought to introduce in a simple form before I launch into more complex discussions. One of these is the concept of a survival distribution.
A probability distribution is a type of function that describes the behavior certain types of random events. Most gamers should intuitively understand the probabilities that result from a single roll of a die, which is formally known as the Discrete Uniform distribution, and those with a basic knowledge of statistics will be familiar with the Normal distribution.
Survival distributions are a subset of probability distributions that can describe the probability that some length of time T will pass before an event X occurs. In the discrete case, this becomes the number of attempt or trials T until event X is observed. An example of the latter is the Geometric distribution. Here there is some probability p that the event will occur on each trial. A simple example would be: “How many times will you roll a six-sided die before getting “6” as the result. The probability of getting a “6” is p=1/6 = 0.1667, and the average or expected value is 1/p or six trials (rolls of the die) before getting a six.
 The other reason for this post (the truth comes out) is that I had a nice plot left over from my previous example using the game Hammer’s Slammers, but really needed to describe the Geometric distribution first. Here is a chart illustrating the distribution of the number of attacks on a unit before it is destroyed. For example, a unit subject to 6 1:1 attacks has about 35% chance of survival (or 65% chance of destruction). As you can see, the lower attack-defense ratios (1-3 to 1-2) might require a large number of attempts before destroying the unit, but the higher ratios have very good change of destroying the target in just a few (or one) attempts.
The other reason for this post (the truth comes out) is that I had a nice plot left over from my previous example using the game Hammer’s Slammers, but really needed to describe the Geometric distribution first. Here is a chart illustrating the distribution of the number of attacks on a unit before it is destroyed. For example, a unit subject to 6 1:1 attacks has about 35% chance of survival (or 65% chance of destruction). As you can see, the lower attack-defense ratios (1-3 to 1-2) might require a large number of attempts before destroying the unit, but the higher ratios have very good change of destroying the target in just a few (or one) attempts.So that, in a nutshell, is my introduction to survival distributions. In the ongoing discussion of the value of units within a game, this is going to be a key concept; the longer a unit survives within a game, the more opportunity there will be for it to be useful - to destroy the other players units - and the longer it can survive the greater its value will be. Next up (well, soon) will be an introduction to a type of survival distribution that can apply to Point Objective Games.
UPDATE: See the next post in this series.
28 November 2008
Anatomy of a Black Hawk H - Part III
 |
| From GBR: Anatomy of a Blackhawk H |
 |
| From GBR: Anatomy of a Blackhawk H |
27 November 2008
Posting policy
- Any posts or links which require any significant effort or my part to determine if they may or may not be legitimate comments, or otherwise wastes my time, are subject to immediate deletion.
- If you want to promote your own blog*, do so by posting a relevant comments, or risk wasting my time (see rule #1). Promote yourself by participating.
- No p@rn. No links to p@rn (see rule #1 again). If you have a relevant comment that includes a link to adult content, then it would be polite to indicate your link is NSFW, Adult, etc.
- If you post in some language other than English, please give some indication of what language that is, or use an automatic translation service such as Google translate. I will not attempt to translated messages if there is no indication that it might be legitimate (see rule #1).
- Be polite. Do not feed trolls. Do not be a troll.
Please note that comments on posts more than 14 days old are subject to moderation, and will not appear immediately.
These rules are subject to my interpretation and may change at any time. If you think you have not been fairly treated, email me your concern and I will consider it.
26 November 2008
General(s) George from Le Petit Soldier Shop
At 528 Royal Street, we made a nice find: The Sword And Pen / Le Petit Soldier Shop. They specialize in historical miniatures and toy soldiers, both modern and antique. Historical miniatures are not my main interest, but I really didn't want to leave this shop empty handed.
---
 |
| From GBR: Public |
 |
| From GBR: Public |
---
 |
| From GBR: Public |
---
I wish I'd had my camera to take some pictures of the displays, but now at least I have an excuse to go back.
23 November 2008
Netrek: The Greatest Star Trek Game

 Last year on a dark November evening I stumbled across an old computer game, one that I had never heard of before. Little did I know that I was going to be spending a fair portion of my free time dueling with other players for control of the galaxy! NETREK is a multiplayer online game loosely based on the Star Trek universe. Up to 16 Players battle each other in 2-D ship-to-ship dogfights, and team play starts when there are enough players for 4-8 players per team (depending on the server). NETREK also has a unique distinction, not only is it a progenitor of modern online games (versions have been around since the mid 1970's), but it was the first game to really take advantage of the online multiplayer setting, making teams and team play the best part of the game.
Last year on a dark November evening I stumbled across an old computer game, one that I had never heard of before. Little did I know that I was going to be spending a fair portion of my free time dueling with other players for control of the galaxy! NETREK is a multiplayer online game loosely based on the Star Trek universe. Up to 16 Players battle each other in 2-D ship-to-ship dogfights, and team play starts when there are enough players for 4-8 players per team (depending on the server). NETREK also has a unique distinction, not only is it a progenitor of modern online games (versions have been around since the mid 1970's), but it was the first game to really take advantage of the online multiplayer setting, making teams and team play the best part of the game.
 The game itself is amazingly simple; Right-Click to change course, left click to fire phasers, "t" for torpedos, "0-9" to set speed, etc. You can learn the basics of play and rudimentary "dogfighting" skills very quickly, and there are practice servers populated with "bots" to aid you in this. Of course, basic skills won't be enough to save you from others who may have been playing for years, but they will let you begin to understand the greater part of the game - the part that transcends the Eighties-tech simplicity of the game. This is because dogfighting is only how you play against other players, it is not the goal of the game.
The game itself is amazingly simple; Right-Click to change course, left click to fire phasers, "t" for torpedos, "0-9" to set speed, etc. You can learn the basics of play and rudimentary "dogfighting" skills very quickly, and there are practice servers populated with "bots" to aid you in this. Of course, basic skills won't be enough to save you from others who may have been playing for years, but they will let you begin to understand the greater part of the game - the part that transcends the Eighties-tech simplicity of the game. This is because dogfighting is only how you play against other players, it is not the goal of the game.
 The goal of NETREK is to transfer armies about between planets and so take control of all the other team's planets, thus winning the game. In a game full of players who all have the basic skill and more, this becomes amazingly difficult. Teams must struggle to accumulate and defend the armies needed to "take" another planet. They must cooperate to mount a successful attack and "take", and then fiercly defend the prize from counter-attack, all the while defending their other planets for similar attacks. To do so successfully requires a level of understanding and cooperation beyond far beyond the "twitchy" skills of dogfighting. Team play arises out of the simple mechanics of the games, transforming it from a simple shooter into a strategic masterpiece as complex as any sport.
The goal of NETREK is to transfer armies about between planets and so take control of all the other team's planets, thus winning the game. In a game full of players who all have the basic skill and more, this becomes amazingly difficult. Teams must struggle to accumulate and defend the armies needed to "take" another planet. They must cooperate to mount a successful attack and "take", and then fiercly defend the prize from counter-attack, all the while defending their other planets for similar attacks. To do so successfully requires a level of understanding and cooperation beyond far beyond the "twitchy" skills of dogfighting. Team play arises out of the simple mechanics of the games, transforming it from a simple shooter into a strategic masterpiece as complex as any sport.Unfortunately, life started making other demands on my time, and my time for NETREK became very limited. Then there was the lightning strike in the spring that fried various bits of electronics about the house, including the network card on the computer I use for games, which ended my NETREK play entirely. Winter is coming around again though, and with it I'll be spending more time indoors looking for something to do. I now have a brand new computer, and I hear there is a new and improved NETREK client available - I think I'll give it a try.
---ADDENDUM---
I also grabbed this image from the NETREK site, which didn't quite fit with the rest of the post. It is a full screen shot from an older version of the Netrek client, and if you click this link, it will take you to an animated GIF of some NETREK action.

19 November 2008
Hammer's Slammers Math (Take 2)
I tried this post before, but I decided I really wanted to do a better job of it. The game Hammer's Slammers has point value for units to aid in creating scenarios, not unlike Battletech's Battle Value. Many games have such point-value systems, but this Hammer's Slammers game is particularly suitable for the sort of simple examples I need to get started. The entire games rules are only about 5-6 pages, and the portion that deal with resolution of combat is maybe half of than. My main goal is to demonstrate a statistical way of looking at the basic mathematics of the game. I'll start off with a bit more about how the game actually plays, or at least the detail of how combat is resolved. Here is the description of the games counters, and if you have any familiarity with typical wargames then this is pretty standard. This particular counter represents a Supertank, and is the most powerful unit in the game. It has attack and defense factors of 8 each, a range of 4 hexes (8 hexes at 1/2 attack factor) and can move 6 hexes per turn.
Here is the description of the games counters, and if you have any familiarity with typical wargames then this is pretty standard. This particular counter represents a Supertank, and is the most powerful unit in the game. It has attack and defense factors of 8 each, a range of 4 hexes (8 hexes at 1/2 attack factor) and can move 6 hexes per turn. To the right is the Combat Results Table (CRT) from Hammer's Slammers as I have recreated it. The attacker declares which units (within range) will attack the defending unit(s) in a single hex (stacking limit is 3). They sum up the attack factors of all attacking and defending units, then find the column that is closest to the ratio of attack to defense factors (rounding down), then roll 2d6 and look up the result. I am mostly interested in the probability of "Dis" and "DE" results, and so will ignore the additional results related to turning hexes to rubble.
To the right is the Combat Results Table (CRT) from Hammer's Slammers as I have recreated it. The attacker declares which units (within range) will attack the defending unit(s) in a single hex (stacking limit is 3). They sum up the attack factors of all attacking and defending units, then find the column that is closest to the ratio of attack to defense factors (rounding down), then roll 2d6 and look up the result. I am mostly interested in the probability of "Dis" and "DE" results, and so will ignore the additional results related to turning hexes to rubble.
An example may be helpful: An attacker with one Supertank (attack factor 8) is attacking a Combat Car (defense factor 4). The ratio is 8 to 4, or 2 to 1. The attacker rolls 2d6 and gets an 8. Looking this up on the "2-1" column of the CRT gives the result "Dis". The Combat Car is disrupted and the counter is flipped over to indicate this. If the Combat Car had already been disrupted, this result would destroy it. If the attacker had rolled a 9 instead of an 8 it would have been destroyed outright.
In this game most Infantry units are never disrupted, and are only affected by "DE" results. The exceptions are not relevant for this discussion.
 There are two probabilities to be considered. The first if the probability that an attack either Disrupts or Destroys the defending unit. The second is the probability the defending units is Destroyed. These probabilities are represented in the chart at the right with the Attack-Defense ratio on the X-axis and the probability on the Y-axis. (Actually, we might also consider the probability of a unit being Disrupted and not destroyed, but I'll save that for another day.) These probabilities are basic to understanding the game, but this is not the only way they can be represented.
There are two probabilities to be considered. The first if the probability that an attack either Disrupts or Destroys the defending unit. The second is the probability the defending units is Destroyed. These probabilities are represented in the chart at the right with the Attack-Defense ratio on the X-axis and the probability on the Y-axis. (Actually, we might also consider the probability of a unit being Disrupted and not destroyed, but I'll save that for another day.) These probabilities are basic to understanding the game, but this is not the only way they can be represented. This is the same graph except the Y-axis now represents Odds instead of probability. If the probability of an event is equal to P, then the Odds of the event are P/(1-P). I have fudged a little bit where the probability that a unit is either Disrupted or Destroyed is equal to 1.0; here the odds are undefined (divide by zero) and I have arbitrary substituted of an odds of 90 so I could have complete graphs here. While probabilities are between zero and one, the odds range from zero to infinity, and large values are not uncommon. It is often useful to consider the odds on a different scale of measurement.
This is the same graph except the Y-axis now represents Odds instead of probability. If the probability of an event is equal to P, then the Odds of the event are P/(1-P). I have fudged a little bit where the probability that a unit is either Disrupted or Destroyed is equal to 1.0; here the odds are undefined (divide by zero) and I have arbitrary substituted of an odds of 90 so I could have complete graphs here. While probabilities are between zero and one, the odds range from zero to infinity, and large values are not uncommon. It is often useful to consider the odds on a different scale of measurement. Now here is a different scale. The Y-axis now represent the natural log of the odd (log-odds), and the X-axis is log-base-2 of the attack-defense ratio. This is an odd decision of my part to use two different logarithmic scales. I chose log-base-2 for the X-axis simply because the graph looks nicer: "0.0" is at zero on this scale, and each doubling (or halving) of the A/D ratio is a shift of 1.0 on the log-2 axis. Otherwise, there is no reason to used log-base-2 instead of the natural log.
Now here is a different scale. The Y-axis now represent the natural log of the odd (log-odds), and the X-axis is log-base-2 of the attack-defense ratio. This is an odd decision of my part to use two different logarithmic scales. I chose log-base-2 for the X-axis simply because the graph looks nicer: "0.0" is at zero on this scale, and each doubling (or halving) of the A/D ratio is a shift of 1.0 on the log-2 axis. Otherwise, there is no reason to used log-base-2 instead of the natural log.Note that the relationships between the log-odds and log A-D ratio can be approximately represented by a straight line, or by the equation of a line, such as
Log(Odds) = A*Log(A/D) + B,
where A and B are coefficients that define the line.
Representing this relationship by the equation of a line is easily accomplished by use of linear regression - a basic statistical technique with a multitude of applications. The implication I am (finally!) getting at, is that there is a statistical way to describe the relationship between attack factor, defense factor, and the probability of a unit being destroyed (or disrupted).
There is more to consider: In a typical game units may be attacked more than once before being destroyed (and in Battletech this is a certainty). To look at what happens when a unit is subjected to a sequence of attacks I will need to discuss survival distributions. Stay tuned.
17 November 2008
Crisis = Danger + Opportunity

There is a common saying that the Chinese symbol for "Crisis" is a combination of the symbols for "Danger" and "Opportunity". While this is not really true, the underlying truth about the nature of crisis remains. We currently have a global financial crisis that represents a danger and opportunity for many businesses, which brings me to today's announcement from Catalyst Game Labs:
Catalyst Game Labs has tendered an offer with The Topps Company, Inc. to acquire various WizKids’ properties, including such dynamic game lines as HeroClix, the Pirates Pocket Model Game, BattleTech/MechWarrior, and Shadowrun. After carefully reviewing options, Catalyst firmly believes that to protect the properties and ensure the best possible continuity for all communities, acquiring all brands will ensure the on going success of these game lines.
I agree. Catalyst is the obvious choice to pick up and carry these game lines, and this represents a great opportunity for them. It is also a danger, and I am concerned that these are difficult times for Catalyst to be taking on greater financial responsibility. Economic hardship hits everyone, and especially the player base of these games, many of whom are young adults without much expendable income to start with. When money is hard to come by, games are going to be pretty low down on everyone's priority list.
So Catalyst faces opportunity and danger, but is the business to be gained worth the business risk at this time? I'm going to hazard my opinion: YES.
This is only my opinion, and I don't have any sort of special information to back it up, but here is my reasoning: The people who own and run Catalyst are highly motivated and enthusiastic about what they do. Creating games isn't a day job for most (any?) of these folks. They work very hard at their jobs, and then they work harder at the games they love. These folks are also busy. Really busy. There are always pressing deadlines and jobs to do. Normally adding yet more to manage might be a bad thing, but managing these new properties seems to be such a natural fit here that I think it can only help them. The remaining question in my mind is "how much is it going to cost them?", and here Catalyst seems to be in a great spot, because if the licensing rights cost too much they can simply walk away no worse than they were before.
There is perhaps one more obvious point to mention; people at Catalyst obviously thought enough of this opportunity to publicly announce they are negotiating with Topps, which may indicate they are already close to a deal. I wish them the best of luck. More: I'll be cheering for them too!
[UPDATE - 12/16/2006]
Catalyst and Pinata Games are teaming up. Maybe. And who is Pinata Games anyway?
16 November 2008
Anatomy of a Blackhawk H - Part II
 I applied a white primer and rough green base coat (Vallejo Game Color Dark Green) . I actually did that last session, but had already put the camera away.
I applied a white primer and rough green base coat (Vallejo Game Color Dark Green) . I actually did that last session, but had already put the camera away.---

I evened out the base coat with more Vallejo Dark Green with some black (Vallejo Model Color Glossy Black) mixed in where I want a darker base tone. I thin out my paints with blending medium and extender to get the consistency and working time I like.
I haven't put the paint on so thick where I intend to put the "stripes" because that will get painted over anyway. Not that you can tell from this picture.
It's about at this point that a little voice in my head started telling me:
Ignoring this little voice is one of the hardest things I have to do, but some of my best work has come out of these jobs that started out looking so very wrong, and that little voice yelling in my ear. I haven't done much blending in green-tones though, a lot of which will be needed to make this work, so I'm a bit uncertain of myself at this point.
GAWD that is ugly! You should drop it in the Pine Sol and start over!
----
 Some time later --> The blue stripes are mostly on (but not finished), and the green tones are working out well. I'm beginning to think this might work (The little voice seems to have gone home in disappointment, but it will be back with the next mini). I haven't begun to obsess over the details yet though, and there is a lot more to do.
Some time later --> The blue stripes are mostly on (but not finished), and the green tones are working out well. I'm beginning to think this might work (The little voice seems to have gone home in disappointment, but it will be back with the next mini). I haven't begun to obsess over the details yet though, and there is a lot more to do.---
13 November 2008
Camospecs Diorama, GENCON 2007
I only wish I had made a few notes about this, and factions were taking part in the battle. Maybe if I zoom in ...
[UPDATE: Randall Bills writes - The diorama depicts the battle on Acturus where Word of Blake forces attempted to destroy a coalition force that “Uncle Chandy” had assembled to attack the Ruins of Gabriel (the 2008 diorama). - Except I think he means 2007.]
---
---
---
---
---
---
There is also a video of this from Catalyst Game Labs:
11 November 2008
Wizkids Closes Shop
[date added]Announcement [11/10/008]
The Topps Company announced today that WizKids will immediately cease operations and discontinue its product lines.
Scott Silverstein, CEO of Topps, said “This was an extremely difficult decision. While the company will still actively pursue gaming initiatives, we feel it is necessary to align our efforts more closely with Topps current sports and entertainment offerings which are being developed within our New York office.”
Good news from Topps and Catalyst: CBT is not affected.
10 November 2008
VASSAL
The VASSAL Game EngineVASSAL is a game engine for building and playing online adaptations of board games and card games. It allows users to play in real time over a live Internet connection (in addition to playing by email). It runs on all platforms, and is free for personal use.
This seems like just the thing for running long distance boardgames.
09 November 2008
Dice Wars

Battles are resolved by highest total dice roll (ties to the defender), with a winner take all result. Replacement dice are given based on the number of adjacent territories you control, and are assigned randomly. A sound strategy is to leave paths of weakly defended territories open to your biggest piles of dice, inviting other (computer controlled) players to attack you so that you can counter-attack with greater force. You also want to set up paths for your opponents to fight each other, so they do not spend all their efforts on you.
[Updated 11/10]




